The mathematical statement “19/32 = 5/8” represents a comparison of two fractions. It explores the concept of fractional equivalence, investigating whether two fractions represent the same proportion or value. For instance, dividing both the numerator and denominator of 5/8 by the common factor of 5 results in 1/1.6 This, however, differs from the result of reducing 19/32 to its simplest form, where no common integer divisor exists.
Understanding fractional equivalence is fundamental to arithmetic and algebra. Accurate fraction manipulation is crucial for numerous applications, including engineering calculations, financial modeling, and scientific measurements. Proficiency in this area ensures correct results and avoids errors in more complex computations. A solid grasp of these principles forms the base for more advanced mathematical concepts.
This foundational understanding will be applied throughout this article to explore topics such as simplifying fractions, finding common denominators, and solving equations involving fractions. The principles examined here provide a solid framework for navigating these more complex mathematical operations.
Images References
Source: printableiacobelliol.z14.web.core.windows.net
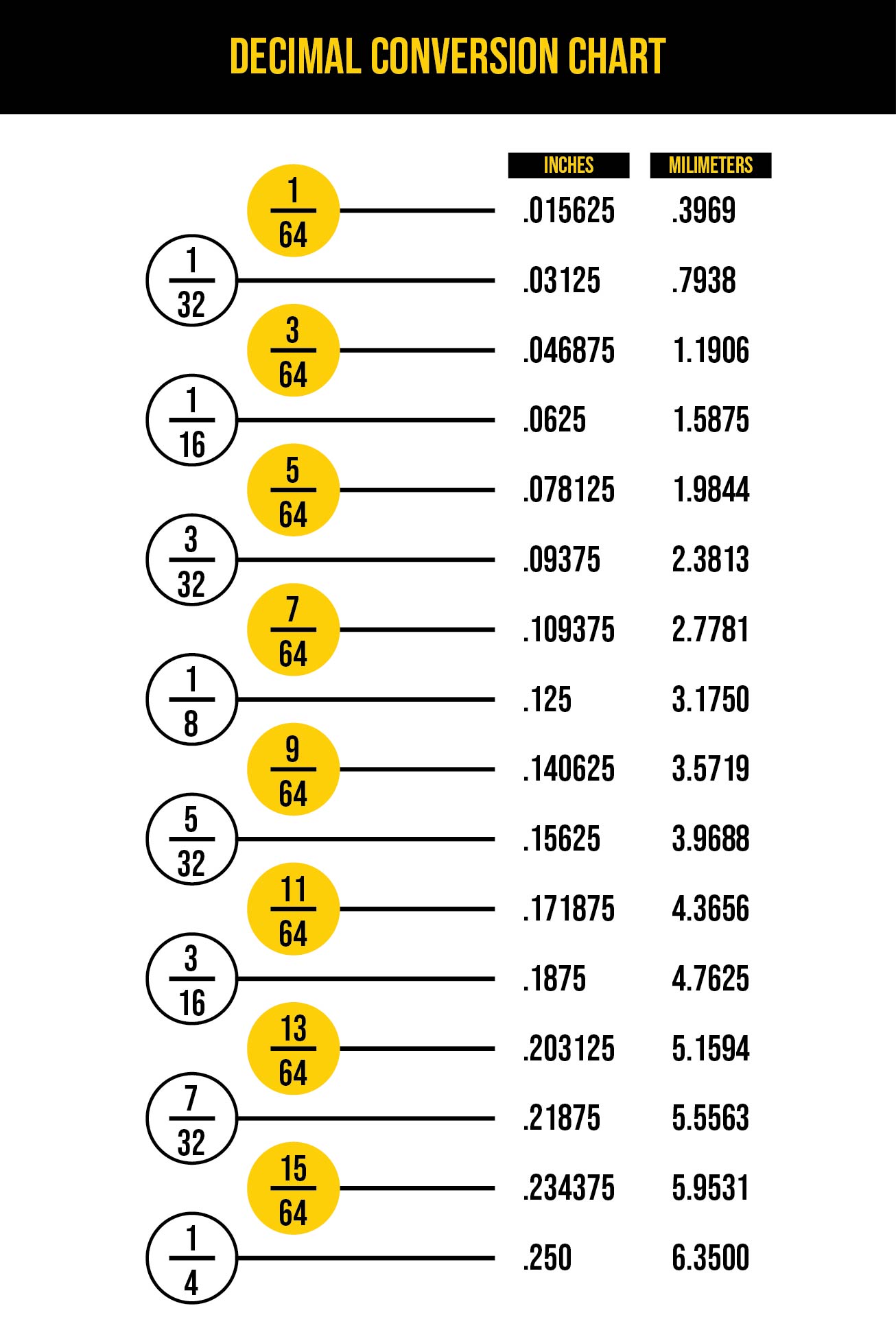
Decimal To Fraction Cheat Sheet
Source: mavink.com
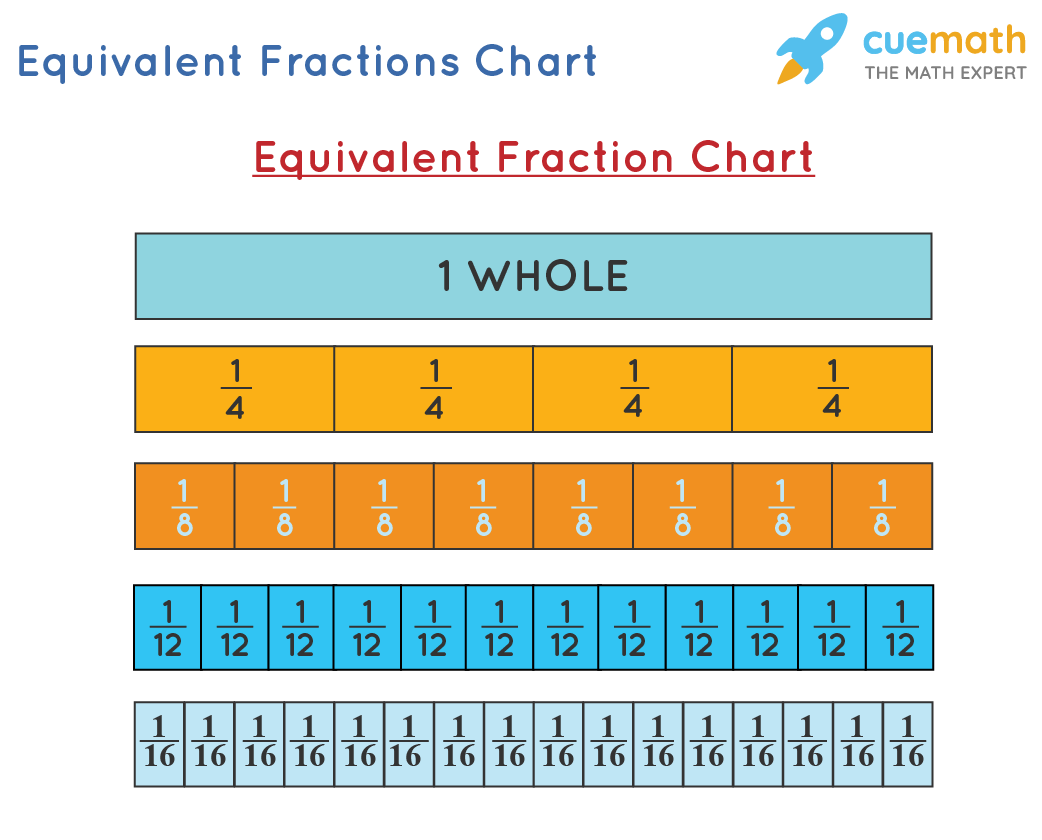
Equivalent Fractions Chart
Leave a Reply